Corso
Inizio 12 febbraio 2021
Michele Brun e Giorgio Carta (DIMCM, Università degli Studi di Cagliari)
Teachers
- Michele Brun, DIMCM, Università degli Studi di Cagliari
- Giorgio Carta, DIMCM, Università degli Studi di Cagliari
Hours: 20
Start date: 12 February 2021

Abstract
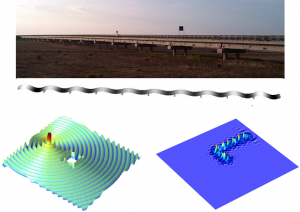
Metamaterials are artificially engineered media, possessing unique properties that cannot be found in nature. In this course, we will discuss the fundamentals of the theory for the modelling and design of mechanical metamaterials, consisting of periodic arrangements of elastic structural elements. In particular, we will focus the attention on the dynamic properties of periodic systems, both discrete (e.g. lattices of masses connected by springs) and continuous (like rods, beams and plates). We will show how the concept of a “phononic crystal” can be used in the design of a real structure, such as a bridge. Furthermore, we will describe the special effect induced by introducing gyroscopic spinners or designed resonating elements into systems of beams and plates. Finally, we will show several classes of possible applications in the field of mechanical metamaterials.
Course description
Structures made of repetitive units are very common in different engineering fields. Bridges, pipelines and skyscrapers are some examples of periodic structures that are widely used for civil applications. The dynamic behaviour of these media exhibits superior attenuation and wave guiding properties. For this reason, they are often referred to as “mechanical metamaterials”.
The course presents the theoretical background of wave propagation in periodic elastic systems. The concepts of dispersion relation, pass- and stop-bands, group and phase velocities are discussed in the framework of linear waves. The analytical approach is applied to both discrete and continuous systems. It is also shown how the properties of periodic systems are linked to the natural frequencies and vibration modes of finite structural elements.
Important practical applications are described. In particular, it is explained how the natural frequencies of a real bridge can be predicted by means of an analytical model, based on the theory of periodic structures. Moreover, it is shown how the introduction of gyroscopic spinners into an elastic structure can affect its dynamic behaviour and how propagation of waves inside the system can be controlled. A presentation of the most advanced applications in the field of mechanical metamaterials will conclude the course.
The course is addressed to graduate and doctoral students in civil and mechanical engineering, applied mathematics and physics, academic and industrial researchers, who are interested in structural dynamics. A basic knowledge of mechanics of solids and structures is recommended.
The use of a mathematical computation software, such as Matlab or Mathematica, is desirable during the practical sessions.
Programme
- 12/02/21, 9:00 – 10:00 (1 hour) [MB]
Introduction on mechanical metamaterials (lecture for a wide audience)
- 12/02/21, 10:00 – 12:00 (2 hours) [GC]
Finite discrete systems: eigenfrequencies and eigenmodes of SDOF and MDOF oscillators
- 15/02/21, 9:00 – 11:00 (2 hours) [GC]
Periodic discrete systems: Bloch-Floquet waves, dispersion relation and transfer matrix
- 15/02/21, 11:00 – 13:00 (2 hours) [GC]
Lab: Determination of the dispersion properties of a bi-atomic lattice
- 16/02/21, 9:00 – 12:00 (3 hours) [MB]
Finite continuous systems: eigenfrequencies and eigenmodes of rods, beams and plates
- 16/02/21, 12:00 – 13:00 (1 hour) [MB]
Lab: calculation of eigenfrequencies and eigenmodes of beams with specific boundary conditions
- 17/02/21, 9:00 – 12:00 (3 hours) [MB]
Periodic continuous systems: Bloch-Floquet waves, dispersion relation, Green’s function and transfer matrix
- 18/02/21, 9:00 – 11:00 (2 hours) [GC]
Design of a bridge as a phononic structure
- 18/02/21, 11:00 – 13:00 (2 hours) [GC]
Elastic systems with gyroscopic spinners: directional localisation and control
- 19/02/21, 9:00 – 11:00 (2 hours) [MB]
Mechanical metamaterials: platonic crystals with inertial resonators, invisibility cloaks
Contacts
mbrun@unica.it, giorgio_carta@unica.it
070 675 5701
Enrollment
To enrol to the course, please send an email to the teachers.
Materials
The notes and slides will be made available to the attendants of the course.
Bibliography and references
Textbooks:
- Brillouin L., “Wave Propagation in Periodic Structures – Electric Filters and Crystal Lattices”, Second Edition, Dover Publications, Inc., 1953.
- Graff K.F., “Wave Motion in Elastic Solids”, Second Edition, Dover Publications, Inc., New York, 1991.
Selected papers:
- Romeo F. and Luongo A., “Invariants representation of propagation properties for bi-coupled periodic structures”, Journal of Sound and Vibration 257, 869-886, 2002.
- Carta G. and Brun M., “Bloch-Floquet waves in flexural systems with continuous and discrete elements”, Mechanics of Materials 87, 11-26, 2015.
- Carta G., Giaccu G.F. and Brun M., “A phononic band gap model for long bridges. The Brabau bridge case”, Engineering Structures 160, 66-76, 2017.
- Carta G., Jones I.S., Movchan N.V., Movchan A.B. and Nieves M.J., “‘Deflecting elastic prism’ and unidirectional localisation for waves in chiral elastic systems”, Scientific Reports 7, 26, 2017.
- Garau M., Nieves M.J., Carta G. and Brun M., “Transient response of a gyro-elastic structured medium: Unidirectional waveforms and cloaking”, International Journal of Engineering Science 143, 115-141, 2019.
- Morvaridi, M., Carta, G. and Brun, M., “Platonic crystal with low-frequency locally-resonant spiral structures: wave trapping, transmission amplification, shielding and edge waves”, Journal of the Mechanics and Physics of Solids 121, 496-516, 2018.
- Brun, M., Guenneau, S. and Movchan, A.B., “Achieving control of in-plane elastic waves” Applied Physics Letters 94, 061903, 2009.
- Colquitt, D.J., Jones, I.S., Movchan, N.V., Movchan, A.B. Brun and M., McPhedran, R.C., “Making Waves Round a Structured Cloak: Lattices, Negative Refraction and Fringes” Proceedings of the Royal Society of London A: Mathematical, Physical and Engineering Sciences 469, 2157, 20130218, 2013.

